Count by 103 (Skip Counting by 103s)

Count by 103

Here we will show you how to count by 103, discuss counting by 103 patterns, and tell you why knowing how to count by 103 matters. To start off, note that Count by 103 means counting in 103s, or count by one hundred threes, and it is also called skip counting by 103.

How to count by 103
Normally, we would count by 1 like this: 1, 2, 3, 4, etc., but when we count by 103, we count 103, 206, 309, 412, and so on.

In other words, to count in intervals of 103 or skip counting by 103, we start with 103 and then add 103 to get the next number, and then continue adding 103 to the previous number to keep counting by 103, like this:

103
103 + 103 = 206
206 + 103 = 309
309 + 103 = 412
412 + 103 = 515
...

You can of course skip count by 103 forever, so it is impossible to make a list of all numbers, but below is a Count by 103 Chart of the first 100 numbers to get you started.

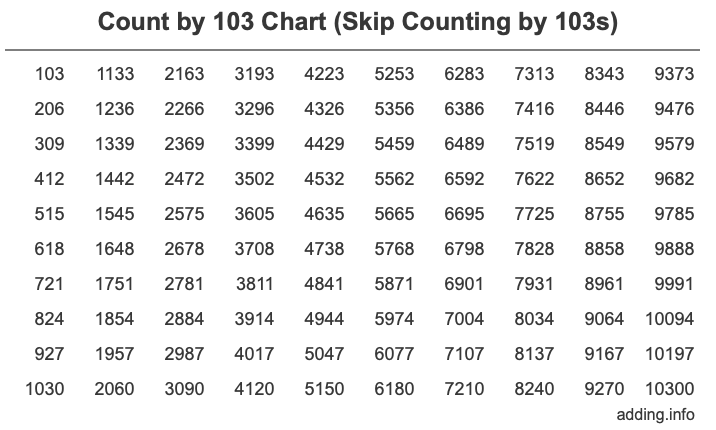
Looking at the chart above, you will see that the first column has the first ten numbers you get when you skip count by 103, the second column has the next ten numbers you get when you skip count by 103, and so forth.

Count by 103 Patterns
We organized the Skip Counting by 103s Chart above in 10 rows and 10 columns so you can easily identify patterns.

Skip counting always creates patterns. Figuring out these patterns may help you if want to count by 103, but don't have the Counting by 103s Chart above. Obviously, one pattern with counting by 103s is that the number increases by 103.

Furthermore, if you look at each row above, each number in the row has the same last digit (ones place). That means that every tenth number has the same last digit.

If you look down the columns, you will see that the last digit (ones place) repeats itself in blocks of 10 over and over. The pattern of the last digit when you count by 103 goes 3, 6, 9, 2, 5, 8, 1, 4, 7, 0 and 3, 6, 9, 2, 5, 8, 1, 4, 7, 0 and so on for as long as you count by 103.

Why Count by 103?
We think that understanding and learning about skip counting by 103 is important, because it teaches you how the arithmetic operations fit together. Below are some examples of what we mean.

When you count by one hundred three, you are also creating a list of multiples of 103 that you can use in math when you need the least common multiple. 103 times n equals the nth multiple or skip count of 103.

When you skip count by 103, you are also creating a list of numbers that 103 is divisible by. On top of that, skip counting by 103 is the same as making the 103 times table.

Skip Counting
Need to skip count by another number? Enter another number for us to skip count for you.

Count by 104
Here is the next number on our list that we used to skip count.