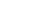To find the answer to "What two numbers have a product of 92 and a sum of 50?" we first state what we know. We are looking for x and y and we know that x • y = 92 and x + y = 50.
Before we keep going, it is important to know that x • y is the same as y • x and x + y is the same as y + x. The two variables are interchangeable. Which means that when we create one equation to solve the problem, we will have two answers.
To solve the problem, we take x + y = 50 and solve it for y to get y = 50 - x. Then, we replace y in x • y = 92 with 50 - x to get this:
x • (50 - x) = 92
Like we said above, the x and y are interchangeable, therefore the x in the equation above could also be y. The bottom line is that when we solved the equation we got two answers which are the two numbers that have a product of 92 and a sum of 50. The numbers are:
1.91321
48.08679
That's it! The two numbers that have a product of 92 and a sum of 50 are 1.91321 and 48.08679 as proven below:
1.91321 • 48.08679 ≈ 92
1.91321 + 48.08679 = 50
Note: Answers are rounded up to the nearest five decimals if necessary so the answers may not be exact.
Product Sum Calculator
Need the answer to a similar problem? Submit another product and sum below to find the two numbers that make your product and sum.